Annu. Rev. Astron. Astrophys. 1998. 36: 435-506 Copyright © 1998 by Annual Reviews. All rights reserved

2. THE CENSUS OF LOCAL GROUP DWARFS

In 1971, Paul Hodge wrote an influential review in these volumes devoted to dwarf galaxies. The total dwarf population of the Local Group at that time was 14 galaxies, including the Magellanic Clouds, with six additional uncertain cases. As we see below, the current census of likely dwarf members of the Local Group now stands at 38_-2⁺⁶. More dwarfs have been confirmed or identified as Local Group members in the past 27 years than during the previous 222 years beginning with Le Gentil's discovery of M32! And it is almost certain that the full census of Local Group dwarfs is not yet complete (Section 2.2).

Merely making a list of Local Group dwarf galaxies demands reliable distances and radial velocities, as well as agreement on the definition of a dwarf galaxy. Table 1 lists all the galaxies that I consider potential Local Group members, without regard to whether they may be dwarf or "giant" galaxies. Also listed are their Hubble types (van den Bergh 1994a), with some modifications and additions for galaxies discovered since that paper. M31, the Milky Way, and M33 are normal "giant" spirals, and I do not consider them in any detail in this review. The Magellanic Clouds - particularly the Small Magellanic Cloud - have a more valid claim to dwarfhood, but I do not discuss them here either. Both have been the subject of recent comprehensive reviews (Olszewski et al 1996b, Westerlund 1990, 1998). All remaining galaxies listed in Table 1 are considered "dwarfs" for the purposes of this review.

2.1. Membership in the Local Group

RESOLVABILITY AND THE BRIGHTEST STARS     A common method of flagging possible Local Group members is to identify "resolved" galaxies with low heliocentric velocities. This has been useful to confirm the proximity of candidate Local Group members; some recent examples include galaxies studied by Hoessel et al (1988; EGB 0427+63), Lavery & Mighell (1992; Tucana), and Whiting et al (1997; Antlia). A quantitative variation of this technique is to identify and measure the brightest red and blue stars in a resolved system (Sandage 1986b;, Sandage & Carlson 1982, 1985a, b, 1988). Piotto & Capaccioli (1992), Rozanski & Rowan-Robinson (1994) reassessed this technique, and both concluded that it is a crude distance indicator ( > 0.5 mag in the distance modulus) in the optical and the infrared (IR). In contrast, Karachentsev & Tikhonov (1993), Lyo & Lee (1997) concluded that the systematic uncertainties are considerably smaller: ~ 0.4 mag in the optical and even smaller in the K band for the brightest red stars (Lyo & Lee 1997). In view of the wide range of star-formation histories exhibited by Local Group dwarfs (Section 6) and these contradictory conclusions, the brightest blue and red stars should be used with care as distance indicators. However, this approach clearly remains suitable for identifying possible Local Group members worthy of further study.

DISTANCES     Table 2 lists the latest information on distances for all the dwarf members and candidates of the Local Group. It is satisfying that nearly all of the galaxies listed now have reasonable distance determinations based on one or more high-precision distance indicator, including (a) Cepheid variables (e.g. Madore & Freedman 1991, Capaccioli et al 1992, Piotto et al 1994, Saha et al 1996, Wilson et al 1996), (b) the I-band tip of the red giant branch (TRGB) (e.g. Da Costa & Armandroff 1990, Lee et al 1993c, Aparicio 1994, Aparicio et al 1997b), (c) RR Lyr stars (Saha & Hoessel 1990, Saha et al 1990, 1992a, b), (d) SX Phe stars (McNamara 1995, Mateo et al 1998b), and (e) the luminosity of the horizontal branch (HB) (Ibata et al 1994, Smecker-Hane et al 1994, Caputo et al 1995, Da Costa et al 1996). Other indicators that serve for more luminous galaxies such as supernovae, surface brightness fluctuations, Tully-Fisher (TF), or D_n - relations are either inapplicable or remain uncalibrated for very low-luminosity dwarfs. Since many individual Local Group galaxies have distances determined with independent techniques, an analysis similar to that of Huterer et al (1995) - which seeks simultaneous consistency of all distance indicators in all galaxies considered - would be of great interest. Generally, when two or more groups have studied the same galaxy, the agreement of CCD-based results is excellent.

Table 2. Distances and heliocentric velocities of Local Group galaxies

Galaxy	lb	bc	E(B-V)	(m-M)0	Distance	Reff	V,opt		V,radio	Refi
namea			magd	mage	(kpc)		(km s-1)g		(km s-1)h
WLMj	75.9	-73.6	0.02 ± 0.01	24.83 ± 0.08	925 ± 40	1–3, 135	—		-123 ± 3	4, 5
NGC 55j	332.7	-75.7	0.03 ± 0.02	25.85 ± 0.20	1480 ± 150	8, 9	—		124 ± 6	4, 8, 136, 137
IC 10	119.0	-3.3	0.87 ± 0.12	24.58 ± 0.12	825 ± 50	10, 11	-344 ± 5		-342 ± 6	4, 5, 12–14
NGC 147	119.8	-14.3	0.18 ± 0.03	24.30 ± 0.12	725 ± 45	1, 15	-193 ± 3		—	16, 22
And IIIk	119.3	-26.2	0.05 ± 0.02	24.40 ± 0.10	760 ± 40	18	—		—	4, 19
NGC 185	120.8	-14.5	0.19 ± 0.02	23.96 ± 0.08	620 ± 25	1, 20	-210 ± 7		-204 ± 4	16, 21–25
NGC 205l	120.7	-21.7	0.04 ± 0.02	24.56 ± 0.08	815 ± 35	1, 26, 27	-242 ± 3		-229 ± 5	4, 16, 22, 29–31,
										138
M32	121.2	-22.0	0.08 ± 0.03	24.53 ± 0.08	805 ± 35	32	-197 ± 3		—	4, 34, 157
M31	121.2	-21.6	0.08	24.43	770	159		-297		159
And Ik	121.7	-24.9	0.04 ± 0.02	24.53 ± 0.10	805 ± 40	36, 37	—		—	4, 19
SMC	302.8	-44.3	0.08	18.82	58	125, 159		-175		159
Sculptork,m	287.5	-83.2	0.02 ± 0.02	19.54 ± 0.08	79 ± 4	39, 40, 145	108 ± 3		102 ± 5	4, 41, 42, 44,
										142
LGS 3	126.8	-40.9	0.08 ± 0.03	24.54 ± 0.15	810 ± 60	45, 151–153	-282 ± 4		-272 ± 6	4, 46, 155
IC 1613	129.8	-60.6	0.03 ± 0.02	24.22 ± 0.10	700 ± 35	1, 27, 47, 48	-237 ± 5		-234 ± 3	4, 49–51, 156
And II	128.9	-29.2	0.08 ± 0.02	23.6 ± 0.4	525 ± 110	52	—		—
M33	133.6	-31.3	0.08	24.62	840	159		-181		159
Phoenixm	272.2	-68.9	0.02 ± 0.01	23.24 ± 0.12	445 ± 30	53, 55	—		56 ± 3/-23 ± 2	54, 144
Fornaxk	237.1	-65.7	0.03 ± 0.01	20.70 ± 0.12	138 ± 8	56	53 ± 3		—	4, 57, 142
EGB 0427+63	144.7	-10.5	0.30 ± 0.15	25.6 ± 0.7	1300 ± 700	61, 146, 147	—	324	-87 ± 6	4
LMC	280.5	-32.9	0.06	18.45	49	125, 159				159
Carinak	260.1	-22.2	0.04 ± 0.02	20.03 ± 0.09	101 ± 5	63–66, 148	224 ± 3		—	67–69
Leo An	196.9	+52.4	0.01 ± 0.01	24.2 ± 0.3	690 ± 100	70, 141, 161	—		26 ± 2	4, 5, 46, 139
Sextans B	233.2	+43.8	0.01 ± 0.02	25.64 ± 0.15	1345 ± 100	71	—		303 ± 2	4, 46, 50, 152
NGC 3109j	262.1	+23.1	0.04 ± 0.02	25.48 ± 0.25	1250 ± 165	1, 72–75, 143	—		404 ± 2	4, 76, 77
Antlia	263.1	+22.3	0.05 ± 0.03	25.46 ± 0.10	1235 ± 65	78, 79, 158	—		361 ± 2	80
Leo Ik	226.0	+49.1	0.01 ± 0.01	21.99 ± 0.20	250 ± 30	81, 82	286 ± 2		—	4, 83, 84, 142
Sextans A	246.2	+39.9	0.03 ± 0.02	25.75 ± 0.15	1440 ± 110	71, 86	328 ± 5		325 ± 3	4, 49, 87
Sextansk	243.5	+42.3	0.03 ± 0.01	19.67 ± 0.08	86 ± 4	88–90	227 ± 3		—	44, 91–93
Leo IIk	220.2	+67.2	0.02 ± 0.01	21.63 ± 0.09	205 ± 12	95–97	76 ± 2		—	4, 98, 142
GR 8	310.7	+77.0	0.02 ± 0.02	26.0 ± 0.7	1590 ± 600	100–102, 162	—		215 ± 3	4, 46, 50, 154
Ursa Minork	105.0	+44.8	0.03 ± 0.02	19.11 ± 0.10	66 ± 3	103, 104	-248 ± 2		—	105, 106, 142
Dracok	86.4	+34.7	0.03 ± 0.01	19.58 ± 0.15	82 ± 6	108, 160	-293 ± 2		—	105, 109, 142
Milky Way	0.0	0.0	—	14.52	8	125	—		—
Sagittariusk,o	5.6	-14.1	0.15 ± 0.03	16.90 ± 0.15	24 ± 2	111–114, 149	140 ± 5		—	111, 115, 116,
SagDIG	21.1	-16.3	0.22 ± 0.06	25.2 ± 0.3	1060 ± 160	117–119	-75 ± 5		-79 ± 2	4, 119, 144
NGC 6822j	25.3	-18.4	0.26 ± 0.04	23.45 ± 0.15	490 ± 40	1, 2	-53 ± 4		-54 ± 6	4, 49, 121–123,
										136
DDO 210n	34.0	-31.3	0.06 ± 0.02	24.6 ± 0.5	800 ± 250	120, 124, 125	—		-137 ± 3	4, 5, 46
IC 5152	343.9	-50.2	0.01 ± 0.02	26.01 ± 0.25	1590 ± 200	126, 134	—		124 ± 3	4, 5
Tucanap	322.9	-47.4	0.00 ± 0.02	24.73 ± 0.08	880 ± 40	127–129	—		—	132
UKS2323-326	11.9	-70.9	0.07 ± 0.03	25.6 ± 0.5	1320 ± 350	118	—		62 ± 6	119
Pegasuso	94.8	-43.5	0.02 ± 0.01	24.90 ± 0.10	955 ± 50	130, 131, 150	—		-182 ± 2	4, 5, 46, 144
aNames in italic are "giant" galaxies, included here for convenience but not discussed in any detail in this review.
b, c Galactic longitude and latitude, respectively.
d Foreground reddening.
e True distance modulus.
f Distance/reddening references: 1, Lee et al 1993c; 2, Gallart et al 1996c; 3, Minniti & Zijlstra 1996; 4, Huchtmeier & Richter 1986; 5, Richter et al 1987; 6, Heisler et al 1997; 7, Côté 1995; 8, Puche et al 1991; 9, Pritchett et al 1987; 10, Wilson et al 1996; 11, Saha et al 1996; 12, Saito et al 1992; 13, Wilson & Reid 1991; 14, Shostak & Skillman 1989; 15, Han et al 1997; 16, Bender et al 1991; 18, Armandroff et al 1993; 19, Thuan & Martin 1979; 20, Lee et al 1993b; 21, Held et al 1992; 22, Young & Lo 1997a; 23, Wiklind & Rydbeck 1986; 24, Welch et al 1996; 25, Ford et al 1977; 26, Lee 1996; 27, van den Bergh 1995; 29, Carter & Sadler 1990; 30, Held et al 1990; 31, Peterson & Caldwell 1993; 32, Grillmair et al 1996; 33, deleted in proof; 34, Dressler & Richstone 1988; 36, Da Costa et al 1996; 37, Mould & Kristian 1990; 39, Schweitzer et al 1995; 40, Da Costa 1984; 41, Queloz et al 1995; 42, Armandroff & Da Costa 1986; 44, Carignan et al 1998; 45, Lee 1995b; 46, Lo et al 1993; 47, Saha et al 1992a; 48, Huterer et al 1995; 49, Tomita et al 1993; 50, Hoffman et al 1996; 51, Lake & Skillman 1989; 52, König et al 1993; 53, Ortolani & Gratton 1988; 54, Carignan et al 1991; 55, van de Rydt et al 1991; 56, Beauchamp et al 1995; 57, Mateo et al 1991b; 59, Karachentsev & Tikhonov 1993; 60, deleted in proof; 61, Hoessel et al 1988; 62, deleted in proof; 63, Smecker-Hane et al 1994; 64, Hurley-Keller et al 1998; 65, Mighell 1997; 66, McNamara 1995; 67, Mateo et al 1993; 68, Godwin & Lynden-Bell 1987; 69, Mould et al 1990; 70, Hoessel et al 1994; 71, Piotto et al 1994; 72, Lee 1993; 73, Capaccioli et al 1992; 74, Musella et al 1997; 75, Richer & McCall 1992; 76, Jobin & Carignan 1990; 77, Carignan 1985; 78, Whiting et al 1997; 79, Aparicio et al 1997c; 80, Fouqué et al 1990; 81, Lee et al 1993a; 82, Demers et al 1994a; 83, Mateo et al 1998c; 84, Zaritsky et al 1989; 86, Sakai et al 1996; 87, Skillman et al 1988; 88, Mateo et al 1995a; 89, Mateo et al 1991a; 90, Irwin et al 1990; 91, Hargreaves et al 1994a; 92, Suntzeff et al 1993; 93, Da Costa et al 1991; 95, Mighell & Rich 1996; 96, Demers & Irwin 1993; 97, Lee 1995b; 98, Vogt et al 1995; 100, Tolstoy et al 1995; 101, Wyatt & Dufour 1993; 102, Aparicio et al 1988; 103, Nemec et al 1988; 104, Olszewski & Aaronson 1985; 105, Armandroff et al 1995; 106, Hargreaves et al 1994b; 108, Nemec 1985; 109, Hargreaves et al 1996b; 111, Ibata et al 1994; 112, Mateo et al 1995b; 113, Alard 1996; 114, Alcock et al 1997; 115, Ibata et al 1997; 116, Koribalski et al 1994; 117, Longmore et al 1978; 119, Longmore et al 1982; 120, Marconi et al 1990; 121, Gottesman & Wiliachew 1977; 122, Israel 1997; 123, Wilson 1994b; 124, Greggio et al 1993; 125, van den Bergh 1994a; 126, Caldwell et al 1988; 127, Saviane et al 1996; 128, Da Costa 1994a; 129, Castellani et al 1996; 130, Aparicio 1994; 131, Hoessel et al 1990; 132, Oosterloo et al 1996; 133, Richter et al 1991; 134, Burstein & Heiles 1982; 135, Hodge & Miller 1995; 136, Israel et al 1995; 137, Hummel et al 1986; 138, Sage & Wrobel 1989; 139, Young & Lo 1996a; 140, deleted in proof; 141, Demers et al 1984; 142, Knapp et al 1978; 143, Davidge 1993; 144, Young & Lo 1997b; 145, Kaluzny et al 1995; 146, Karachentseva et al 1996; 147, Karachentsev et al 1994; 148, Mateo et al 1998b; 149, Sarajedini & Layden 1995; 150, Aparicio et al 1997b; 151, Aparicio et al 1997a; 152, Mould 1997; 153, Tikhonov & Makarova 1996; 154, Carignan et al 1990; 155, KH Cook, EW Olszewski, C Stubbs, private communication; 156, Lu et al 1993; 157, Nolthenius & Ford 1986; 158, Sarajedini et al 1997; 159, Karachentsev & Makanov 1996; 160, Grillmair et al 1998; 161, Tolstoy et al 1998; 162, Dohm-Palmer et al 1998.
g Heliocentric velocity based on optical measurements.
h Heliocentric velocity based on radio measurements.
iV elocity references; see footnote f.
j Photographic spectra were obtained by Humason et al (1956), from which they measured velocities of -78, 210, and 441 km s-1 for WLM, NGC 55, and NGC 3109, respectively. The typical uncertainty in these results is approximately 50 km s-1.
k No HI detected. See Table 5 for limits.
l HI, CO, and optical velocities differ by small but possibly significant amounts.
m Ambiguous HI detection; the velocity of the most plausible component is listed. For Phoenix, one or both of two HI clouds could be associated with the galaxy (Carignan et al 1991).
n Large distance discrepancy or uncertainty; see text.
o All values refer to the central regions of the galaxy, taken to coincide with the globular cluster M54.
p A nearby HI cloud (V ~ 130 km s-1) is observed about 15 arcmin from Tucana, which may be associated with the Magellanic Stream (Oosterloo et al 1996).

There are exceptions. Aparicio (1994) used the TRGB method to argue that the distance of the Pegasus dIrr galaxy is considerably closer than the Cepheid-based distance of Hoessel et al (1990). Aparicio noted that many of the putative Cepheids are located on or near the red giant branch (RGB). Wilson (1994a) identified variables in IC 10 that she tentatively claimed might be Cepheids and proposed a surprisingly small distance for the galaxy. Soon afterwards, Wilson et al (1996), Saha et al (1996) obtained IR and optical photometry of bona fide Cepheids in IC 10 to settle the issue, finding good consistency for D ~ 830 kpc. Hoessel et al (1994) identified five possible Cepheids in Leo A, deriving a distance of 2.2 Mpc. This result is inconsistent with the low velocity of Leo A relative to the Local Group barycenter (Figure 1), implies a pathologically large red-to-blue supergiant ratio (Wilson 1992a), forces the HI mass of Leo A to exceed the kinematic mass of the entire galaxy (Section 7), and results in an abnormally low M/L ratio for the galaxy (Young & Lo 1996). Tolstoy et al (1998) have readdressed this problem using new HST and ground-based photometry, and they concluded that Leo A is ~ 0.8 ± 0.2 Mpc away. This result resolves all of the problems noted above for Leo A.

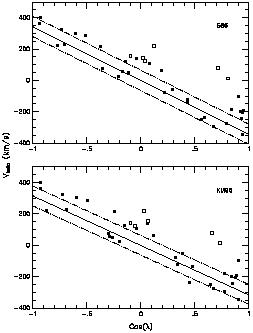
Figure 1. The heliocentric velocities of nearby galaxies plotted as a function of cos(), where is the angle between the galaxy and the location of the apex of the Sun's motion relative to the center of mass of the Local Group. The upper plot adopts the solution for the solar motion from Sandage (1986), for which the apex is located at (l, b) = (101°, -11°) and the Sun's velocity component in this direction is 343 km s-1. The lower plot is for the solution from Karachentsev & Makarov (1996), for which (l,b) = (93°, -4°) and the solar velocity is 316 km s-1. The filled squares denote the galaxies adopted as Local Group members in this paper; the open squares represent a sample of other nearby galaxies - SclDIG (Heisler et al 1997), NGC 300 (Puche et al 1990), Maffei 1 (Luppino & Tonry 1993), DDO 187, and UGCA 86 (van den Bergh 1994a) - that are shown here as a representative sample of galaxies that have at some time or other been considered to be Local Group members (see van den Bergh 1994a for other examples).

DYNAMICAL CONSIDERATIONS     Yahil et al (1977) introduced a dynamical approach that uses only the observed radial velocities to determine the motions of candidate group members relative to the Local Group barycenter. Sandage (1986a) extended this approach by defining the zero-velocity surface separating the Local Group from the local Hubble expansion field. This method acknowledges that the size and internal dispersion of the Local Group (1.5-2 Mpc in radius and ~ 60 km s^-1, respectively) imply a crossing time comparable to or longer than a Hubble time. Thus, some bound Local Group members may not yet have reached the outer limit of their first orbit and consequently are still receding from the Local Group center of mass. Sandage's approach requires an estimate of the Local Group mass. The current consensus for the mass of the Galaxy has risen since 1986 to about 1.4 ± 0.7 x 10¹² M within 200 kpc from the Galactic Center (Zaritsky et al 1989, Fich & Tremaine 1991, Lin et al 1995, Peebles 1995, Kochanek 1996). If we assume that M31 is 30% more massive than the Milky Way (Peebles 1989), the total mass of the Local Group is M_LG = 3.3 ± 1.6 x 10¹² M; the zero-velocity surface is about 1.8 Mpc from the Local Group barycenter (Sandage 1986a).

A simple variant of this approach that I use here recognizes that for a bound system traveling at velocity v along a radial orbit, the enclosed mass is M_enc 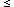v² R / G for a distance R from the barycenter. For radial orbits, heliocentric velocities can be used to estimate the mass of the Local Group for each dwarf galaxy with or without corrections for the Sun's offset from the Local Group barycenter. Galaxies that imply large values of M_enc are probably not bound to the Local Group and are well into the Hubble flow. Using the radial velocities compiled in Table 2, I have calculated M_enc for all Local Group dwarf-galaxy candidates in Table 1. Karachentsev (1996) developed a related method that employs estimates of both the energy and orbital time scales of individual dwarfs to establish membership.

The results of this analysis are shown in Figure 1. Following Yahil et al (1977), I plot the heliocentric velocity, V, vs cos for individual Local Group candidates, where is the angle between the galaxy and the apex of the solar motion relative to the Local Group barycenter. The two panels correspond to the solar-motion solutions of Sandage (1986a), Karachentsev & Makharov (1996; their equation 4). For the most distant galaxies, the correction from heliocentric to barycentric velocity is small. Galaxies for which M_enc exceeds the observed Local Group mass are denoted as open squares. For both solar-motion solutions, NGC 55 - usually considered a member of the Sculptor Group - is consistent with Local Group membership, as are NGC 3109 and the other galaxies near it (see Section 2.3). EGB 0427+63 and GR 8 are marginal Local Group members; both galaxies imply M_enc is slightly larger than the adopted Local Group mass.

Of course, galaxies with large tangential velocities might still be unbound even if their radial velocities relative to the Local Group barycenter are low (Dunn & Laflamme 1993). Proper motions have now been measured (impressively) for some Milky Way satellites: Sculptor (Schweitzer et al 1995), Ursa Minor (AE Schweitzer, KM Cudworth, SR Majewski, in preparation), the Large Magellanic Cloud (LMC) (Jones et al 1994), and Sagittarius (Ibata et al 1997). We can expect to measure the tangential motions of other Local Group galaxies out to ~ 1 Mpc over four- to five-year baselines using HST. For now it is probably not possible to unambiguously determine membership for galaxies near the outer fringe of the Local Group armed with only radial velocities and distances. This is further complicated by the effects that nearby groups must have had on the orbits of galaxies in the outer Local Group (Sandage 1986a, Peebles 1989, 1995).

2.2. What's Missing?

van den Bergh (1995) suggests that about 98% of the luminous mass of the Local Group has already been identified. But have we identified all of the individual galaxies? Since 50% of the members listed in Table 2 have been found since 1971, the era of discovery within the Local Group probably is not yet over. If we assume that the Local Group galaxy distribution is uniform on the sky (though see Section 2.3), the cumulative number of galaxies should increase from the Galactic poles as 1 -sin |b|, where b is Galactic latitude. Figure 2 shows the cumulative number of all Local Group members (top panel) and MW satellites (bottom panel) as a function of 1 - sin |b|. For comparison, the dotted lines show how a uniformly distributed sample would appear in these diagrams. Note that in both samples, the observed number of galaxies exceeds the predicted distribution at high galactic latitude (small values of 1 -sin|b|). This suggests that many Local Group galaxies remain to be found at low latitudes. If I extrapolate from the observed number of galaxies at the fiftieth and sixty-seventh percentile values of 1 -sin|b|(the vertical lines in Figure 2), as many as 15-20 more Local Group galaxies may be hidden at low latitudes, up to half of which may be satellites of the Milky Way. Using the technique of Yahil et al (1977), Grebel (1997) has identified three to four additional Local Group candidates from the catalog of Schmidt & Boller (1992); none of these have even preliminary distance estimates yet. Karachentseva & Karachentsev (1998) have compiled a list of nearby dwarfs that contain additional candidate Local Group members (also see Karachentsev & Makarov 1998).

Figure 2. Plots of the cumulative distribution of all Local Group galaxies (upper plot) and of the galaxies in the MW subgroup (lower plot) as a function of (1 - sin |b|), where b is Galactic latitude. The dotted lines show the expected distribution of a uniform sample of 40 and 12 objects. The vertical lines show where 50% (left) and 67% (right) of a uniform cumulative distribution would be found. For example, based on the fiftieth percentile value of Nc = 10 in the lower panel, a total of 20 MW satellites would be expected if they were uniformly distributed and if there was no gradient in Galactic extinction as a function of latitude.

The recent discoveries of Sextans (Irwin et al 1990) and Sagittarius (Ibata et al 1994) illustrate that nearby galaxies can also hide if they are too diffuse. Caldwell et al (1998) recently found a low-surface brightness, but relatively luminous, dwarf in the M81 group with _0,V = 25.4 mag arcsec^-2 and M_V = -14.3. If this galaxy - which has a core radius of 1.6 kpc - were located 1 Mpc from us, it would contribute 22 stars arcmin^-2 brighter than I = 22 (corresponding to the more luminous giants), or only 0.8 stars arcmin^-2 brighter than I = 18.5 if located 200 kpc away. The apparent core radius would be 5.4 and 27 arcmin, respectively. An object like this would be difficult to identify even at intermediate Galactic latitude (Irwin 1994), though methods designed to find stars far from the centers of known dwarfs might succeed (e.g. Gould et al 1992, Mateo et al 1996, Kuhn et al 1996, Siegel et al 1998).

Henning (1997) summarizes prospects for finding "hidden" galaxies from HI observations at low latitudes. Although the Galaxy is transparent at 21 cm, confusion with Galactic emission at low velocities would present a major obstacle to finding Local Group galaxies in this manner. Of course, only gas-rich objects would be found, effectively ruling out detection of gas-poor early-type dwarfs at low latitudes. Ongoing IR surveys such as DENIS (Epchtein et al 1997) and 2MASS (Kirkpatrick et al 1997) may successfully penetrate much of the foreground dust in some regions of the Galactic Plane. Although the stellar density of Galactic field stars would be quite high, the signature of a nearby dwarf galaxy might be possible to detect as a concentrated excess of faint stars at low latitudes.

2.3. The Structure of the Local Group

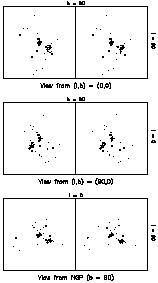
Because they are so numerous, the Local Group dwarfs can be used effectively as tracers of substructure. Gurzadyan et al (1993), Karachentsev (1996) have previously carried out substructure analyses of the Local Group; both found the well-known concentration of galaxies towards M31 and the Milky Way (see also van den Bergh 1995, Grebel 1997). A powerful - but more subjective - way to visualize local substructure is via stereoscopic images of the distribution of Local Group galaxies. Three stereo views are shown in Figure 3a, corresponding to observers located well outside the Local Group along the orthogonal axes (l, b) = (0°, 0°), (90°, 0°), and b = + 90°, centered on the Galactic Center. Figure 3b identifies some of the more distant individual galaxies for each of three viewing positions of

Figure 3. Stereoscopic views of the Local Group based on the data in Tables 1 and 2. From top to bottom, these views are from (l, b) = (0°, 0°), (90°, 0°) and the NGP, respectively. The directions of the principle orthogonal axes in the plane of the paper are indicated on the top and right edges of each stereoscopic pair. The "viewer" is located 2.6 Mpc from the center of the Milky Way, which is represented by the large cross closest to the center of each panel; the other large cross represents M31; and the small cross is M33. Open squares represent the dSph and E galaxies (see Table 1 for galaxy types); closed squares are Irr galaxies; small xs are transition systems (denoted dIrr/dSph in Table 1). To produce the stereo effect, the viewer's "eyes" were set about 250 kpc apart. The MW, M31, and NGC 3109 subgroups are easily seen in all three stereo pairs; the Local Group Cloud is best appreciated in the middle pair but is also evident as a distinct structure in the other two panels. Only GR 8 (and possibly Leo A) is unattached to any subgroup; this is best seen in the lower panel where the galaxy is particularly near the observer. The set of three single panels on the opposite page serve to aid identification of individual galaxies in the stereo pairs. The galaxies closest to M31 and the Milky Way have not been labeled to avoid excessive clutter in the diagram. Because of the strong perspective effects required to make a stereo pair, none of the panels represents orthogonal projections of the galaxy positions onto the various planes.

Four subgroups are evident in Figure 3. The prominent group located near the center is the Milky Way and its satellites (the origin of the coordinate system is the center of our Galaxy). The second surrounds M31. The third forms an extended "cloud" populated only by dwarfs, mostly dIrr systems. This "Local Group Cloud" is best seen in the middle panel of Figure 3a. The fourth subgroup is relatively isolated and contains NGC 3109 as its most luminous member. The individual members of these four subgroups are identified in Table 1. Remarkably few galaxies have ambiguous subgroup assignments: IC 1613 and Phoenix could both be plausibly placed in the MW or M31 subgroups; Leo A's new distance (Tolstoy 1998) places it in an isolated location between the MW and N3109 subgroups. Only GR 8 - a marginal Local Group member - cannot be clearly assigned to any of these four subgroups.

It is interesting that the N3109 subgroup "points" toward the nearby Maffei / IC 342 group (Karachentsev et al 1996), while the Local Group Cloud very roughly points toward the Sculptor group (Côté et al 1997). NGC 55 is located on the far side of the Local Group Cloud, closest to the Sculptor group. Perhaps the substructure of the Local Group reflects a dynamical history in which the Maffei and Sculptor groups have had an important role and have "stretched out" the Local Group in these directions (Byrd et al 1994). Alternatively, this may reflect a false excess of candidates in these directions where the surface densities of galaxies just beyond the Local Group are relatively high. NGC 55 in particular may be an example of this effect.